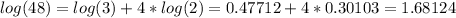Answer:
log(48) = 1.68124
Explanation:
Notice that the number 48 can be written as the product of 3 times 16, and also that 16 can be written as "2" to the power 4:
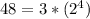
so now we can find the log of 48 using the properties of logarithms;

Since we are given specific values for log(3) [0.47712] and log(2) [0.30103], we can use these values in the expression above to get the numerical answer we are seeking: