Answer:
A. k=29400N/m
B. x=0.528m
Step-by-step explanation:
Part A.
The net force exerted by the driver on the springs is equal to his weight. Assuming the springs reach an equilibrium, we have that this force is equal and opposite in direction to the force exerted by the springs. So, from the equation of the force exerted by a spring, we have:
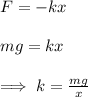
Plugging in the given values for the mass of the driver and the compression lenght, we obtain:
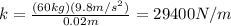
So, the spring constant of the spring system in the taptap is 29400N/m.
Part B.
We can use the same relationship as in the part A, in this case solving for x:
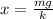
In "m", we have to put the total mass that is in the taptap, so we sum the information given:
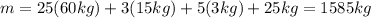
Finally, we calculate x using this value for m:

Then, the springs are compressed 0.528m.