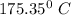Question

Three return steam lines in a chemical processing plant enter a collection tank operating at steady state at 9 bar. Steam enters inlet 1 with flow rate of 1.4 kg/s and quality of 0.9. Steam enters inlet 2 with flow rate of 2 kg/s at 200°C. Steam enters inlet 3 with flow rate of 1.2 kg/s at 95°C. Steam exits the tank at 9 bar. The rate of heat transfer from the collection tank is 40 kW.

Answer 1

Three return steam lines in a chemical processing plant enter a collection tank operating at steady state at 9 bar. Steam enters inlet 1 with flow rate of 1.4 kg/s and quality of 0.9. Steam enters inlet 2 with flow rate of 2 kg/s at 200°C. Steam enters inlet 3 with flow rate of 1.2 kg/s at 95°C. Steam exits the tank at 9 bar. The rate of heat transfer from the collection tank is 40 kW.

Here is the complete part of the question

Neglecting kinetic and potential energy effects.

Determine for the steam exiting the tank.

a) The mass flow rate; in kg/s

b) The temperature in degree C

Answer:

The mass flow rate
`m_4 = 4.6 \ kg/s`

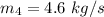
The temperature in degree C =
`175.35^0 \ C`

Step-by-step explanation:

Given that;

At Inlet 1; steam enters with

pressure P₁ = 9 bar

quality x₁ = 0.9

mass m₁ = 1.4 kg/s

At inlet 2;

P₂ = 9 bar

T₂ = 200° C

m₂ = 2.0 kg/s

At inlet 3;

P₃ = 9 bar

T₃ = 95° C

m₃ = 1.2 kg/s

Steam exits with pressure P₄ = 9 bar

Heat transfer
`Q_(cv)` = 40 kW (out)

Now, let's first calculate the mass flow rate of the outlet using mass rate balance for the streams.

`m_1 +m_2 +m_3 = m_4`

Replacing 1.4 kg/s for
`m_1`; 2.0 kg/s for
`m_2`; 1.2 kg/s for
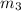`m_3` in the above equation; Then:

`m_4` = (1.4 + 2.0 + 1.2) kg/s

`m_4` = 4.6 kg/s

Thus, the mass flow rate in kg/s = 4.6 kg/s

Using the Table A-3 " properties of saturated water - pressure table to obtain below properties at pressure of 9 bar

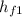`h_(f1)` = 742.56 kJ/kg

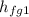`h_(fg1)` = 2030.5 kJ/kg

At section (1) ; the enthalpy can be calculated as:

`h_1= h_(f1) + x_1h_(fg1)`

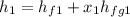
= 742.6 kJ/kg + ( 0.9 × 2030.5 kJ/kg)

= 742.6 kJ/kg + 1827.45 kJ/kg

= 2570.01 kJ/kg

Using the table A - 4 " properties of super-heated vapor to obtain below properties at pressure of 9 bar & 200° C

`h_2 = 2834.1 \ kJ/kg`

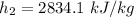
Using the Table A - 4 " properties of saturated water (liquid - vapor ): Temperature Table" to obtain below properties at temperature of 95° C

`h_3 = 398.71 \ kJ/kg`

The formula for the steady flow energy is expressed as:

`Q_(cv ) + m_1h_1+m_2h_2+m_3h_3-m_4h_4 = 0`

`h_4 = \frac{Q_(cv) + m_1h_1+m_2h_2+m_3h_3} {m_4}`

`h_4 = (\frac{- 40 + (1.4*2570.01)+(2.0*2834.1)+(1.2*398.71)} {4.6})`

`h_4 = \frac{- 40 + (3598.014)+(5668.2)+(478.452)} {4.6}`

`h_4 = \frac{9704.666} {4.6}`

`h_4 =2109.71 \ kJ/kg`

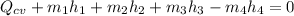
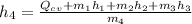
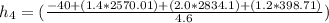
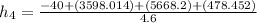
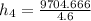
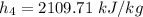
Using the Table A-3 properties of saturated Water-Pressure Table" to obtain below properties at pressure 9 bar

`h_(f4) = \ 742.56\\h_(g4) = 2773.0`

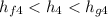`h_(f4) < h_4< h_(g4)` So
`T_4 = T_(sat) \ at \ 9 \ bar`

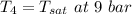
Using the table A - 3 " properties of saturated vapor (liquid - vapor ) : pressure table" to obtain the below properties at pressure = 9 bar

`T_(sat)= T_4 = 175.35^0 \ C`

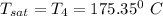
Therefore, The temperature in degree C =
`175.35^0 \ C`

Answer 2

Step-by-step explanation:

Neglecting kinetic and potential energy effects, determine for the steam exiting the tank: (a) the mass flow rate, in kg/s. (b) the temperature, in °C.

The solution is shown in the picture below