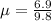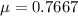Answer:
The coefficient of static friction is
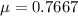
Step-by-step explanation:
From the question we are told that
The acceleration is
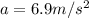
The mathematical expression that show the relationship between frictional force and the sliding force of the cabinet at the point just before sliding begins is
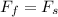
Now
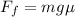
Where m is the mass of the cabinet
g is the acceleration due to gravity
 is the coefficient of static friction
is the coefficient of static friction
And

Where a is the acceleration due to gravity
Now substituting this into the equation
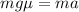
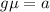
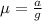
Substituting values where