Question

Super Bowl XLVI was played between the New York Giants and the New England Patriots in Indianapolis. Due to a decade-long rivalry between the Patriots and the city's own team, the Colts, most Indianapolis residents were rooting heartily for the Giants. Suppose that 90% of Indianapolis residents wanted the Giants to beat the Patriots. What is the probability that from a sample of 200 Indianapolis residents, fewer than 170 were rooting for the Giants in Super Bowl XLIV?

Answer 1

Probability that from a sample of 200 Indianapolis residents, fewer than 170 were rooting for the Giants in Super Bowl XLVI is 0.02385.

Explanation:

We are given that Due to a decade-long rivalry between the Patriots and the city's own team, the Colts, most Indianapolis residents were rooting heartily for the Giants. Suppose that 90% of Indianapolis residents wanted the Giants to beat the Patriots.

Let p = % of Indianapolis residents wanted the Giants to beat the Patriots = 90%

The z-score probability distribution for proportion is given by;

Z =
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }` ~ N(0,1)

where,
`\hat p` = % of Indianapolis residents who were rooting for the Giants in Super Bowl XLVI in a sample of 200 residents =
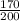`(170)/(200)` = 0.85

n = sample of residents = 200

So, probability that from a sample of 200 Indianapolis residents, fewer than 170 were rooting for the Giants in Super Bowl XLVI is given by = P(
`\hat p` < 0.85)

P(
`\hat p` < 0.85) = P(
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }` <
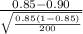`\frac{0.85-0.90}{\sqrt{(0.85(1-0.85))/(200) } }` ) = P(Z < -1.98) = 1 - P(Z
`\leq` 1.98)

= 1 - 0.97615 = 0.02385

The above probability is calculated using z table by looking at value of x = 01.98 in the z table which have an area of 0.97615.

Therefore, probability that fewer than 170 were rooting for the Giants in Super Bowl XLVI is 0.02385.