Question

The differential equation below models the temperature of an 87°C cup of coffee in a 17°C room, where it is known that the coffee cools at a rate of 1°C per minute when its temperature is 67°C. Solve the differential equation to find an expression for the temperature of the coffee at time t. (Let y be the temperature of the cup of coffee in °C, and let t be the time in minutes, with t = 0 corresponding to the time when the temperature was 87°C.) dy dt = − 1 50 (y − 17)

Answer 1

To solve the given differential equation dy/dt = -1/50(y - 17) for the temperature of the coffee at time t, we can use the method of separation of variables. The expression for the temperature of the coffee at time t is y = A
`e^{(-t/50)` + 17.

Step-by-step explanation:

To solve the given differential equation dy/dt = -1/50(y - 17), we can use the method of separation of variables. Let's start by rearranging the equation:

dy/(y - 17) = -dt/50

Now, integrate both sides of the equation:

∫(dy/(y - 17)) = ∫(-dt/50)

ln|y - 17| = (-t/50) + C, where C is the constant of integration.

Next, solve for y by applying the natural logarithm property:

`y - 17 = e^(((-t/50)) + C)\\y - 17 = e^((-t/50)) * e^C\\y - 17 = Ae^((-t/50)), where A = e^C`

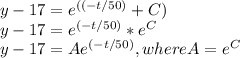
Finally, solve for y to obtain the expression for the temperature of the coffee at time t:

y = A
`e^{(-t/50)`+ 17

Answer 2

y(t) = 17+70e^(-t/50)

Step-by-step explanation: The model of equation given is:

dy/dt = -1/50 (y-17)

Integrate both sides

dy/(y-17) = -1/50 dt

This lead to

ln(y-17) = -1/50 t + C

Log both sides:

y = c e^(-t/50) + 17 ....... (1)

and let t be the time in minutes, with t = 0 corresponding to the time when the temperature was 87°C.

87 = c e^0 + 17

e^0 = 1

Therefore c = 87-17 = 70

y(0) = 87, so c=70

Substitute c into equation 1. This lead to:

y(t) = 17+70e^(-t/50)