Question

A function f(x, y) is called homogeneous of degree n if it satisfies the equation f(tx,ty) = t n f(x, y) for all t, where n is a positive integer. Show that if f(x, y) is homogeneous of degree n, then x ∂ f ∂ x +y ∂ f ∂ y = n f(x, y). (Hint: Try differentiating: find ∂ ∂t t=1 of a homogeneous function f in two ways.)

Answer 1

It is proved that
`x(\partial f)/(\partial x)+y(\partial f)/(\partial y)=nf(x,y)`.

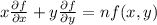Explanation:

Given function is,

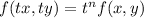`f(tx, ty)=t^nf(x, y)` for all t>0 and n is positive integers.

To show,

`x(\partial f)/(\partial x)+y(\partial f)/(\partial y)=n f(x, y)`

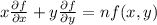
Let, u=tx and v=ty, then,

`f(tx, ty)=t^nf(x, y)`

`\implies f(u, v)=t^n f(x, y)\hfill (1)`

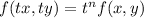
differentiate partially with respect to x we get,

[tex}\frac{\partial}{\partial x}f(u,v)=\frac{\partial}{\partial x}(t^nf(x, y))[/tex]

`\implies(\partial f)/(\partial u)(\partial u)/(\partial x)=t^n(\partial f)/(\partial x)`

`\implies(\partial f)/(\partial u)t=t^n(\partial f)/(\partial x)`

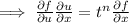
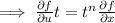
Again, differentiate (1) with respect to t we get,

`(\partial)/(\partial t)f(u,v)=nt^(n-1)f(x,y)`

`\implies (\partial f)/(\partial u)(\partial u)/(\partial t)+(\partial f)/(\partial v)(\partial v)/(\partial t)=nt^(n-1)f(x,y)`

`\implies x(\partial f)/(\partial u)+y(\partial f)/(\partial v)=nt^(n-1)f(x,y)`

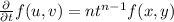
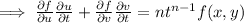
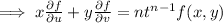
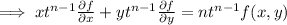`\implies xt^(n-1)(\partial f)/(\partial x)+yt^(n-1)(\partial f)/(\partial y)=nt^(n-1)f(x, y)` (By putting values of
`(\partial f)/(\partial u),(\partial f)/(\partial v)`)

`\therefore x(\partial f)/(\partial x)+y(\partial f)/(\partial y)=nf(x,y)`

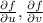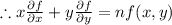
Hence proved.