Question

A new test to detect TB has been designed. It is estimated that 88% of people taking this test have the disease. The test detects the disease in 97% of those who have the disease. The test does not detect the disease in 99% of those who do not have the disease.

If a person taking the test is chosen at random, what is the probability of the test indicating that the person does not have the disease?

a) 0.1452b) 0.9900c) 0.0100d) 0.0300e) 0.0264f) None of the above.

Answer 1

Correct option: (a) 0.1452

Explanation:

The new test designed for detecting TB is being analysed.

Denote the events as follows:

D = a person has the disease

X = the test is positive.

The information provided is:

`P(D)=0.88\\P(X|D)=0.97\\P(X^(c)|D^(c))=0.99`

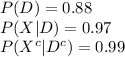
Compute the probability that a person does not have the disease as follows:

`P(D^(c))=1-P(D)=1-0.88=0.12`

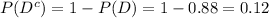
The probability of a person not having the disease is 0.12.

Compute the probability that a randomly selected person is tested negative but does have the disease as follows:

`P(X^(c)\cap D)=P(X^(c)|D)P(D)\\=[1-P(X|D)]* P(D)\\=[1-0.97]* 0.88\\=0.03* 0.88\\=0.0264`

Compute the probability that a randomly selected person is tested negative but does not have the disease as follows:

`P(X^(c)\cap D^(c))=P(X^(c)|D^(c))P(D^(c))\\=[1-P(X|D)]*{1- P(D)]\\=0.99* 0.12\\=0.1188`

Compute the probability that a randomly selected person is tested negative as follows:

`P(X^(c))=P(X^(c)\cap D)+P(X^(c)\cap D^(c))`

`=0.0264+0.1188\\=0.1452`

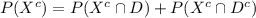
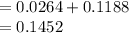
Thus, the probability of the test indicating that the person does not have the disease is 0.1452.