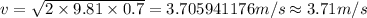Question

g A 2.3 kg block is attached to the spring, and it is released from rest 0.7 m from the spring's equilibrium position. Neglecting friction of the horizontal surface that the block is sliding on, how fast is the block moving once it crosses back by the equilibrium position after being released

Answer 1

3.71 m/s

Step-by-step explanation:

From the law of conservation of linear momentum, since we are neglecting minor energy losses due to friction then we can express it as
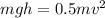`mgh=0.5mv^(2)` since all the potential energy is transformed to kinetic energy

Making v the subject of the formula then
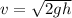`v=\sqrt {2gh}` and here m is the mass of the block, g is acceleration due to gravity, h is the height. Substituting 0.7 m for h and 9.81 for g then we obtain that
`v=\sqrt {2* 9.81* 0.7}=3.705941176 m/s\approx 3.71 m/s`