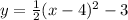Given that the vertex of the parabola is (4,-3)
The parabola passes through the point (2,-1)
We need to determine the standard form of the equation of the parabola.
Standard form of the equation of the parabola:
The standard form of the equation is
 where the vertex is (h,k) and a is the constant.
where the vertex is (h,k) and a is the constant.
Substituting the vertex (4,-3) in the above equation, we get;
 ---------------(1)
---------------(1)
Substituting the point (2,-1) in the above equation, we have;
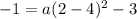
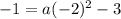
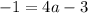
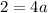
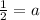
Thus, the value of a is

Substituting the value of a in the equation (1), we get;
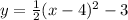
Thus, the standard form of the equation of the parabola is