The value of c is 4 feet for the given triangle it is obtained by law of cosines formula.
Explanation:
The given is,
Triangular diagram with side dimensions
Step:1
Law of Cosines formula is,
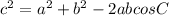 .......................(1)
.......................(1)
From the given diagram,
a = 8 feet
b = 7 feet
Angle, C = 32°
Substitute the values then equation (1) becomes,

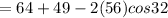
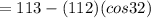
Substitute the value of cos 32°
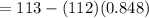 (∵
(∵
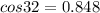 )
)
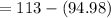
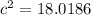
Take square root on both sides the above equation becomes,


c ≅ 4 feet
Result:
The value of c is 4 feet for the given triangle it is obtained by law of cosines formula.