Question

LoL i need help with this question oof

Answer 1

D

Explanation:

5 on the radical is basically power ⅕

[(n+4)³]^⅕

(n+4)^⅗

Answer 2

Given expression:

`\sqrt[5]{(n+4)^(3)}`

To find:

The equivalent expression.

Solution:

`\sqrt[5]{(n+4)^(3)}`

Apply radical rule:

`$\sqrt[m]{a}=a^{(1)/(m)}`

Using this rule in the given expression:

`\sqrt[5]{(n+4)^(3)}=\left((n+4)^(3)\right)^{(1)/(5)}`

Apply exponent rule:

`\left(a^(b)\right)^(c)=a^(b c)`

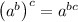
Using this rule:

`\left((n+4)^(3)\right)^{(1)/(5)}=(n+4)^{3 \cdot (1)/(5)}`

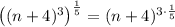
On multiplying 3 and
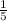`(1)/(5)`, we get
`(3)/(5)`.

`=(n+4)^{(3)/(5)}`

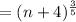
The equivalent expression for the given expression is
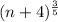`(n+4)^{(3)/(5)}`.