A) height =
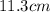 .
.
B) height =
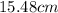 .
.
Explanation:
Here , We need to calculate height of triangles let's do this :
A)
We draw a perpendicular from vertex to base line (8 cm ) , so length becomes 4 cm of base of triangle .
By Pythagoras Theorem we have ,
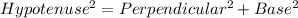
⇒
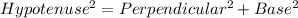
⇒
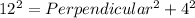
⇒
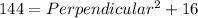
⇒
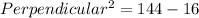
⇒
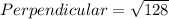
⇒
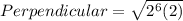
⇒

⇒
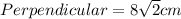
Therefore , height =
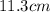 .
.
B)
We draw a perpendicular from vertex to base line (14 cm ) , so length becomes 7 cm of base of triangle .
By Pythagoras Theorem we have ,
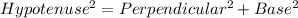
⇒
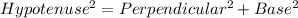
⇒
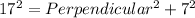
⇒
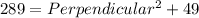
⇒
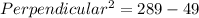
⇒
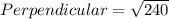
⇒
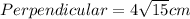
Therefore , height =
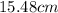 .
.