Explanation:
Logarithm is basically the exponent - also called power - to which a base must be raised to produce a given number.
Mathematically, it can be displayed as:
 is the logarithm of
is the logarithm of
 to the base
to the base
 , if
, if
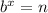 , in which case we write
, in which case we write
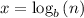
Lets take the first example,

as
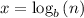
Therefore, 4 is the logarithm of 16 to base 2.
Lets take the second example,
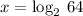
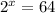
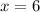
- Logarithmic functions are basically inverse of exponents.
Here is how they are related to each other:
Logarithm → Exponent
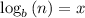 →
→
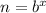
- Logarithms are helpful in displaying large number.