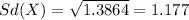Question

Question 7-15 The following probability distribution applies to the high temperature X to be experienced in a city when the morning reading is 24 (in Celsius degrees): x p(x) 30 .05 31 .13 32 .27 33 .36 34 .14 35 .05 Calculate the expected value, variance, and standard deviation for the day's high temperature as expressed in: (a) Celsius degrees expected value of X

Answer 1

a)
`E(X)= 30*0.05 +31*0.13+ 32*0.27 +33*0.36 +34*0.14+ 35*0.05 = 32.56`

b)
`E(X^2) =\sum_(i=1)^n X_i P(X_i)`

And replacing we got:

`E(X^2)= 30^2*0.05 +31^2*0.13+ 32^2*0.27 +33^2*0.36 +34^2*0.14+ 35^2*0.05 = 1061.54`

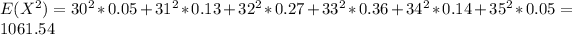
And the variance is given by:

`Var(X)= E(X^2) -[E(X)]^2 = 1061.54- (32.56)^2 = 1.3864`

c)
`Sd(X) = √(1.3864)= 1.177`

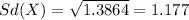
Explanation:

Previous concepts

In statistics and probability analysis, the expected value "is calculated by multiplying each of the possible outcomes by the likelihood each outcome will occur and then summing all of those values".

The variance of a random variable Var(X) is the expected value of the squared deviation from the mean of X, E(X).

And the standard deviation of a random variable X is just the square root of the variance.

Solution to the problem

For this case we have the following probability distribution:

X 30 31 32 33 34 35

P(X) 0.05 0.13 0.27 0.36 0.14 0.05

Part a

We can calculate the expected value with the following formula:

`E(X) =\sum_(i=1)^n X_i P(X_i)`

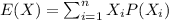
And replacing we got:

`E(X)= 30*0.05 +31*0.13+ 32*0.27 +33*0.36 +34*0.14+ 35*0.05 = 32.56`

Part b

Now we can calculate the second moment with this formula:

`E(X^2) =\sum_(i=1)^n X_i P(X_i)`

And replacing we got:

`E(X^2)= 30^2*0.05 +31^2*0.13+ 32^2*0.27 +33^2*0.36 +34^2*0.14+ 35^2*0.05 = 1061.54`

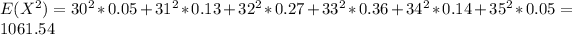
And the variance is given by:

`Var(X)= E(X^2) -[E(X)]^2 = 1061.54- (32.56)^2 = 1.3864`

Part c

And finally the standard deviation is given by:

`Sd(X) = √(1.3864)= 1.177`