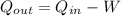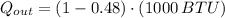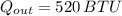Answer:
a)
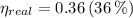 ,
,
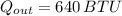 , b)
, b)
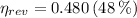 ,
,
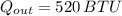
Step-by-step explanation:
a) The efficiency of the reversible power cycle is modelled by the Carnot's cycle:

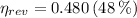
The real efficiency is:

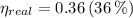
The energy discharged to the cold reservoir is determined herein:
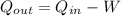

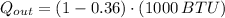
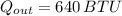
b) The efficiency of the reversible cycle is:
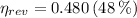
The energy discharge to the cold reservoir is found: