Question

Find the value of the greater root of x² + 10x + 24 = 0.

Answer 1

The greater root is -4.

Explanation:

• The given equation is
  `x^(2) +10x+24 =0`
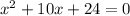
• This a quadratic equation which can be solved using the factorizing method.
• The general form of the quadratic equation is given by
  `ax^(2) +bx +c = 0`

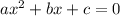
where,

• a is the coefficient of x².
• b is the coefficient of x.
• c is the constant term.

In the given equation, a = 1, b = 10, c = 24.

• Sum of the roots ⇒ b
• Product of the roots ⇒ c

To find the roots of the equation
`x^(2) +10x+24 = 0` :

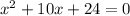• Sum of the roots ⇒ 6+4 = 10 (where b = 10).
• Product of the roots ⇒ 6×4 = 24 (where c = 24).

The solution is given by (x+6)(x+4) = 0.

The roots are given by (x+6) = 0 and (x+4) = 0.

Therefore, x= -6 and x = -4 are the roots of the equation
`x^(2) +10x+24 = 0`

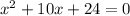
The greater root is -4.