Question

A string is stretched to a length of 339 cm and both ends are fixed. If the density of the string is 0.0073 g/cm, and its tension is 859 N, what is the fundamental frequency?

Answer 1

160 Hz.

Step-by-step explanation:

For nth harmonic, the fundamental frequency,

`f_n=(n)/(2L)\sqrt{(T)/(\mu) }`

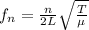
Here T is the tension in string, \mu is the mass/unit of length of the string and L is the string length.

Given n = 1 frequency of the 1st harmonic (the Fundamental), T = 859 N,

L= 339 cm =3.39 m and
`\mu=0.0073 g/cm =0.00073 kg /m`.

Substituting these values, we get

`f_1=(1)/(2*3.39m) \sqrt{(859N)/(0.00073\ kg/m ) }`

`f_1= 0.147 *1084.76=159.99 Hz`

`f_1=160 Hz`

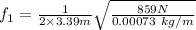
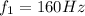
Thus, the fundamental frequency is 160 Hz.