Answer:
Option a) 125 Superscript one-third x is correct
The equivalent expression to the given expression
![\sqrt[3]{125}^x](https://img.qammunity.org/2021/formulas/mathematics/middle-school/km0j9i58uh6ath56xftf67spv322h7xec7.png) is
is

Explanation:
Given that (RootIndex 3 StartRoot 125 EndRoot) Superscript x
Given expression can be written as
![\sqrt[3]{125}^x](https://img.qammunity.org/2021/formulas/mathematics/middle-school/km0j9i58uh6ath56xftf67spv322h7xec7.png)
To find the equivalent expression to the given expression :
![\sqrt[3]{125}^x](https://img.qammunity.org/2021/formulas/mathematics/middle-school/km0j9i58uh6ath56xftf67spv322h7xec7.png)
 ( by using the property
( by using the property
![\sqrt[x]{y}=y^{(1)/(x)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/nith9m23nt2xup8yxthb4kkkv48d01vihn.png) )
)
 ( by using the property
( by using the property
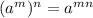 )
)
![\sqrt[3]{125}^x=(125)^{(1)/(3)x}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/xc2sknuxkmgsz1wdv6vzo7c5ulepik0l6l.png)
Therefore the equivalent expression to the given expression
![\sqrt[3]{125}^x](https://img.qammunity.org/2021/formulas/mathematics/middle-school/km0j9i58uh6ath56xftf67spv322h7xec7.png) is
is

Therefore the equivalent expression to the given expression is 125 Superscript one-third x
Therefore option a) 125 Superscript one-third x is correct.