Question

If the sound level at a point is 115 dB, what is the intensity of sound at that point?

(Unit=W/m^2)

Answer 1

The intensity of the sound is
`0.316W/m^2`.

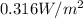Step-by-step explanation:

The equation for the decibel scale is

`dB = 10 \:log((I)/(I_n) )`

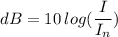
where
`I` is the intensity of sound, and
`I_n = 1*20^(-12)W/m^2` is the intensity of the least audible sound to the human ear.

Now, we are told that at a certain point the sound level is 115dB; therefore,

`115dB = 10 \:log((I)/(1*10^(-12)) )`,

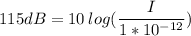which we simplify and solve for
`I`as follows:

`11.5 = log((I)/(1*10^(-12)) )`

`10^(11.5) = (I)/(1*10^(-12))`

`I =1*10^(-12)*10^(11.5)`

`\boxed{I = 0.316W/m^2}`

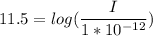
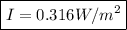
which is the intensity of the sound at the point in question.