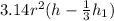Question

Emmanuel carves a cone-shaped hollow out of a solid clay cylinder. What is the approximate volume of the solid portion of the cylinder? Use 3.14 for π.

Answer 1

117in cubed

Hope this helps :)

Answer 2

`3.14r^2(h-(1)/(3)h_1)`

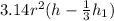
Explanation:

Let h be the cylinders height and r the radius.

-The volume of a cylinder is calculated as:

`V=\pi r^2h`

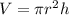
-Since the cone is within the cylinder, it has the same radius as the cylinder.

-Let
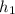`h_1`be the height of the cone.

-The area of a cone is calculated as;

`V=\pi r^2 (h)/(3)\\\\=(1)/(3)\pi r^2h_1`

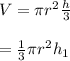
The volume of the solid section of the cylinder is calculated by subtracting the cone's volume from the cylinders:

`V=V_(cy)-V_(co)\\\\=\pi r^2h-(1)/(3)\pi r^2 h_1, \pi=3.14\\\\=3.14r^2(h-(1)/(3)h_1)`

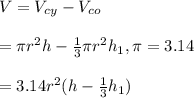
Hence, the approximate area of the solid portion is
`3.14r^2(h-(1)/(3)h_1)`