Question

The radius of the large sphere is double the radius of the small sphere. How many times does the volume of the large sphere than the small sphere? 2 4 6 8

Answer 1

8

Explanation:

E2021

Answer 2

8 times larger.

Explanation:

The radius of the large sphere is double the radius of the small sphere.

Question asked:

How many times does the volume of the large sphere than the small sphere

Solution:

Let radius of the small sphere =
`x`

As the radius of the large sphere is double the radius of the small sphere:

Then, radius of the large sphere =
`2x`

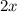
To find that how many times is the volume of the large sphere than the small sphere, we will divide the volume of large sphere by volume of small sphere:-

For smaller sphere:
`Radius = x`

`Volume \ of \ sphere = (4)/(3) \pi r^(3)`

`=(4)/(3) \pi x^(3)`

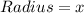
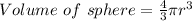
For larger sphere:
`Radius = 2x`

`Volume \ of \ sphere = (4)/(3) \pi r^(3)`

`=(4)/(3) \pi (2x)^(3)`

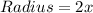
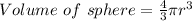
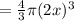
Now, we will divide volume of the larger by the smaller one:

`=(4)/(3) \pi (2x)^(3)/(4)/(3) *(\pi )/(1 ) * x^(3)\\ \\ =(4)/(3) \pi*8x^(3) *(3)/(4\pi )\ *(1)/(x^(3) )`

`(4)/(3)\pi\ is \ canceled\ by\ (3)/(4\pi ) \ and\ also\ x^(3) is\ canceled\ by \ (1)/(x^(3) )`

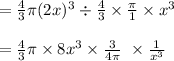
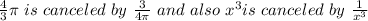
Now, we have

=
`(8)/(1)`

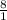
Therefore, the volume of the large sphere is 8 times larger than the smaller sphere.