Answer:
The x-coordinate is changing at 10 cm/s
Explanation:
Rate of Change
Suppose two variables x and y are related by a given function y=f(x). If they both change with respect to a third variable (time, for instance), the rate of change of them is computed as the derivative using the chain rule:
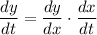
We have
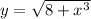
Or, equivalently
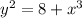
We need to know the rate of change of x respect to t. We'll use implicit differentiation:

Solving for dx/dt
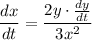
Plugging in the values x=1, y=3, dy/dt=5
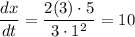
The x-coordinate is changing at 10 cm/s