Question

The residents of a certain dormitory have collected the following data: People who live in the dorm can be classified as either involved in a relationship or uninvolved. Among involved people, 10 percent experience a breakup of their relationship every month. Among uninvolved people, 15 percent will enter into a relationship every month. What is the steady-state fraction of residents who are uninvolved

Answer 1

The steady state proportion for the U (uninvolved) fraction is 0.4.

Explanation:

This can be modeled as a Markov chain, with two states:

U: uninvolved

M: matched

The transitions probability matrix is:

`\begin{pmatrix} &U&M\\U&0.85&0.15\\M&0.10&0.90\end{pmatrix}`

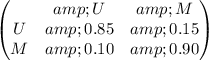
The steady state is that satisfies this product of matrixs:

`[\pi] \cdot [P]=[\pi]`

being π the matrix of steady-state proportions and P the transition matrix.

If we multiply, we have:

`(\pi_U,\pi_M)*\begin{pmatrix}0.85&0.15\\0.10&0.90\end{pmatrix}=(\pi_U,\pi_M)`

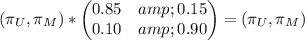
Now we have to solve this equations

`0.85\pi_U+0.10\pi_M=\pi_U\\\\0.15\pi_U+0.90\pi_M=\pi_M`

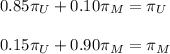
We choose one of the equations and solve:

`0.85\pi_U+0.10\pi_M=\pi_U\\\\\pi_M=((1-0.85)/0.10)\pi_U=1.5\pi_U\\\\\\\pi_M+\pi_U=1\\\\1.5\pi_U+\pi_U=1\\\\\pi_U=1/2.5=0.4 \\\\ \pi_M=1.5\pi_U=1.5*0.4=0.6`

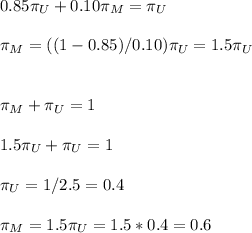
Then, the steady state proportion for the U (uninvolved) fraction is 0.4.