Answer: The rational expression below is undefined when x is equal +5 and -5.
Step-by-step explanation: The rational expression will be undefined when the denominator is equal to zero. So, first you have to take the denominator and make it equal to zero.
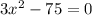
Then you divide the whole thing by three to get this:
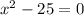
Now you move 25 over to the other side of the equation and take the square-root of both sides.
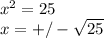
Since the square root of 25 is equal to five. We know that the rational expression will be undefined when x= -5 and +5