Question

What is the image point of (0,4) after a translation right 2 units and down 3 units?

Answer 1

the image point of (0,4) after a translation right 2 units and down 3 units is (2,1) .

Explanation:

Here we have , to find the image point of (0,4) after a translation right 2 units and down 3 units. Let's find out:

Initially we have the point as (0,4) , Following transformations are done :

a translation right 2 units :

The point is (0,4) , let
`(x,y)=(0,4)` . Translation is 2 units right , means there is change in x coordinate by +2 , i.e.

⇒
`(x,y)=(0,4)`

⇒
`(x+2,y)=(0+2,4)`

⇒
`(x+2,y)=(2,4)`

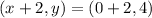
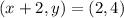
a translation down 3 units:

The point is (2,4) , let
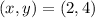`(x,y)=(2,4)` . Translation is 3 units down , means there is change in y coordinate by -3 , i.e.

⇒
`(x,y)=(2,4)`

⇒
`(x,y-3)=(2,4-3)`

⇒
`(x,y-3)=(2,1)`

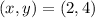
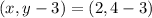
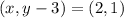
Therefore , the image point of (0,4) after a translation right 2 units and down 3 units is (2,1) .