Answer:
a) v = 21.34 m/s
b) v = 21.34 m/s
c) v = 21.34 m/s
Step-by-step explanation:
Mass of the snowball, m = 0.560 kg
Height of the cliff, h = 14.2 m
Initial velocity of the ball, u = 13.3 m/s
θ = 26°
The speed of the slow ball as it reaches the ground, v = ?
The initial Kinetic energy of the snow ball,

Potential energy of the snow ball at the given height, PE = mgh
Final Kinetic energy of the ball as it reaches the ground,
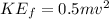
a) Using the principle of energy conservation,
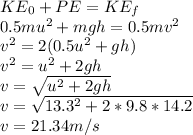
b) The speed remains v = 21.34 m/s since it is not a function of the angle of launch
c)The principle of energy conservation used cancels out the mass of the object, therefore the speed is not dependent on mass
v = 21. 34 m/s