Question

A rock is thrown into a still pond. The circular ripples move outward from the point of impact of the rock so that the radius of the circle formed by a ripple increases at the rate of 4 feet per minute. Find the rate at which the area is changing at the instant the radius is 11 feet.When the radius is 11feet, the area is changing at approximately ____ square feet per minute. (round to the nearest thousandth)

Answer 1

When the radius is 11 feet, the area is changing at approximately 276.571 square feet per minute.

Explanation:

We are given the following in the question:

`(dr)/(dt) = 4\text{ feet per minute}`

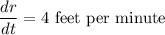
Instant radius = 11 feet

Area of circle =

`A = \pi r^2`

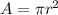
where r is the radius of the circle.

Rate of change of area of circle =

`(dA)/(dt) = (d)/(dt)(\pi r^2) = 2\pi r(dr)/(dt)`

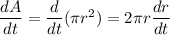
Putting all the values, we get,

`(dA)/(dt) = = 2\pi (11)(4) = 88\pi = 276.571\text{ square feet per minute}`

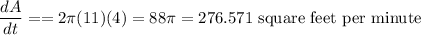
Thus, when the radius is 11 feet, the area is changing at approximately 276.571 square feet per minute.