Answer:
(a) The 90% confidence interval estimate of the percentage of orders that are not accurate in Restaurant A is (0.148, 0.222).
(b) Restaurant B has more proportion of not accurate orders.
Step-by-step explanation:
The (1 - α)% confidence interval for population proportion is:
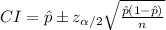
(a)
In Restaurant A the number of not accurate orders was x = 55 of n = 297 orders.
The sample proportion of not accurate orders in Restaurant A is:

The critical value of z for 90% confidence level is:
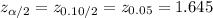
Compute the 90% confidence interval estimate of the percentage of orders that are not accurate in Restaurant A as follows:
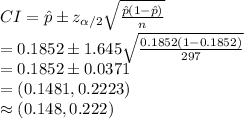
Thus, the 90% confidence interval estimate of the percentage of orders that are not accurate in Restaurant A is (0.148, 0.222).
(b)
The 90% confidence interval estimate of the percentage of orders that are not accurate in Restaurant B is (0.171, 0.245).
The confidence interval for Restaurant B indicates that between 17.1% to 24.5% orders are inaccurate.
The values of this interval is more than that for Restaurant A.
So, it can be concluded that Restaurant B has more proportion of not accurate orders.