Answer:

Step-by-step explanation:
If we consider that all the gravitational potential energy (
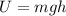 ) gets transformed into thermal energy (
) gets transformed into thermal energy (
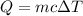 ), we then have:
), we then have:
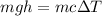
which means:
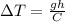
Since the specific heat capacity of water is
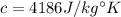 , we have:
, we have:

(A difference in Kelvin is the same difference is Celsius).