Question

9) A construction company employs 2 sales engineers. Engineer 1 does the work in estimating cost for 70% of jobs bid by the company. Engineer 2 does the work for 30% of jobs bid by the company. It is known that the error rate for Engineer 1 is such that 0.02 is the probability of an error when he does the work, whereas the probability of an error in the work of Engineer 2 is 0.04. What is the probability that a bid provided by the company will contain an error in the cost estimates?

If a serious error is known occurred, what is the probability that it was made by Engineer 2?

Answer 1

The probability that the error occurred when Engineer 2 made the mistake is 0.462 on the other hand the probability that the error occurred when the engineer 1 made the mistake is 0.538

Step-by-step explanation:

Let
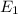`E_(1)` denote the event that the 1st engineer does the work.so we write

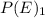`P(E)_(1)`=0.7

Let
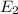`E_(2)` denote the event that the 2nd engineer does the work .So we write

`P(E)_(2)`=0.3

Let O denote the event during which the error occurred .so we write

`P(O/E_(1) )`=0.02(GIVEN)

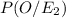`P(O/E_(2) )`=0.04(GIVEN)

• The probability that the error occurred when the first engineer performed the work is
  `P(E_(1) /O)`

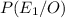
• The probability that the error occurred when the first engineer performed the work is
  `P(E_(2) /O)`

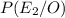
Now we need to find when did the error in the work occur so we will compare the probability of the work done by engineer 1 and engineer 2

lets find the Probability of the Engineer 1

Using Bayes theorem,we get

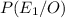`P(E_(1) /O)` =0.02*0.7/0.02*0.7+0.04*0.3 = 0.014/0.026=0.538

lets find the Probability of the Engineer 2

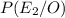`P(E_(2) /O)` =0.04*0.3/0.02*0.7+0.04*0.3=0.012/0.026=0.462

Since ,0.462<0.538 so it is more prominent that the Engineer 1 did the work when the error occurred