Answer :

Step-by-step explanation :
Here, A right angled triangle is given with the measure of two sides and we are to find the measure of the third side.
We'll find the measure of third side with the help of the Pythagorean theorem,
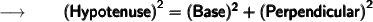
Here,
- The perpendicular (AC) is 5
- The hypotenuse (AB) is c.

So, substituting the values in the formula we get :
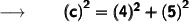
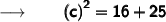


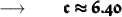
Therefore,
- The measure of the third side (c) is 6.40 .