Answer:
The number of turns in the secondary coil is 28.
Step-by-step explanation:
Given that,
Input voltage given to the transformer,
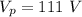
Output voltage supplied to the video game,
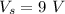
Number of turns in the primary coil,
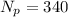
We need to find the number of turns in its secondary coil. We know that the transformer equation is given by :
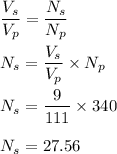
or
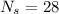
So, the number of turns in the secondary coil is 28.