Answer:
Step-by-step explanation:
Using ohm's law
a) V = IR where V is voltage in Volt, I is current in Ampere and R is resistance in ohms
R = V / I = 1.50 V/ ( 2.05 /1000) A = 731.71 ohms
b) Power = IV =
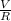 × v =
× v =
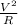 =
=
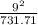 = 0.1107 W
= 0.1107 W
c) E = IR + Ir = ( 731.71 × 0.0036) + ( 35 × 0.0036) = 2.76 V
d) Power use by the resistor = I²R = 0.0036² × 731.71 = 0.00948 W = 0.00948 W = 0.000009483 kw × ( 18 / 60 ) H = 2.84 × 10⁻⁶ KW-H