Question

A manufacturer sells video games with the following cost and revenue functions (in dollars) where x is the number of games sold, for 0 less than or equal to x less than or equal to 3300.

C(x)= 0.32x^2-0.00004x^3
R(x)= 0.848x^2-0.0002x^3

Determine the intervals on which the profit function is increasing.

Answer 1

Therefore the profit interval is (0,2200).

Explanation:

Given cost and revenue function are respectively,

`C(x)= 0.32x^2-0.00004x^3`

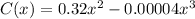
and

`R(x)= 0.848x^2-0.0002x^3`

where x is the number of game sold,
`0\leq x\leq 3300`.

Therefore the profit function is

P(x)= R(x)-C(x)

`=0.848x^2-0.0002x^3-(0.32x^2-0.00004x^3)`

`=0.848x^2-0.0002x^3-0.32x^2+0.00004x^3`

`=0.528x^2 -0.00016x^3`

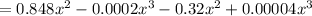
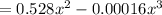
To determine the profit interval where profit function increasing, we have to find the critical point of P(x). We set the first order derivative equal to 0.

Therefore,

`P'(x) = 1.056 x - 0.00048 x^2 =0`

⇒x(1.056-0.00048 x) =0

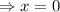`\Rightarrow x = 0` or,
`x= (1.056)/(0.00048) =2200`

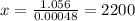
Now choose two point one is less than 2200 and other is greater than of 2200.

We choose x= 1000 and x= 2000

Now

`P'(1000) = 1.056 * 1000- 0.00048 (1000)^2 = 576` >0

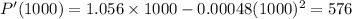`P'(2000) = 1.056 * 2000- 0.00048 (2000)^2 = -1152` <0

Therefore the profit interval is (0,2200).