Answer:
4.6 feet
Explanation:
The equation is:
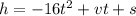
Where
v is initial velocity (given as 16)
s is initial height (given as 0.6)
Substituting, we can write:

This is a quadratic equation of the general form:
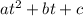
Which we can conclude the coefficients to be:
a = -16
b = 16
c = 0.6
The max height occurs at the value:
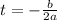
So, max height occurs at:

We will get the max height if we put t = 0.5 into the original equation. So that would be:
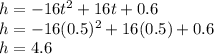
Max Height = 4.6 feet (occurs at t = 0.5 seconds)