Answer:
a. [6.6350,7.3950]
b. ME=0.5150
Explanation:
a. Given that n=40,
 and that:
and that:
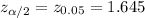
The required 90% confidence interval can be calculated as:
![\bar x\pm(margin \ of \ error)\\\\\bar x\pm z_(\alpha/2)(\sigma)/(√(n))\\\\6.88\pm(1.645* (1.98)/(√(40)))\\\\=[6.3650,7.3950]](https://img.qammunity.org/2021/formulas/mathematics/college/r6n89koagd316ovgkmg4ol3q2qljsmd4sm.png)
Hence, the 90% confidence interval for the population mean cash value of this crop is [6.6350,7.3950]
b. The margin of error at 90% confidence interval is calculated as:
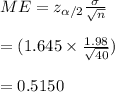
Hence, the margin of error is 0.5150