statement is true!
Explanation:
Here we have , The circle
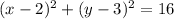 intersect y-axis. We need to find that this statement is true or false . We have the following equation of circle :
intersect y-axis. We need to find that this statement is true or false . We have the following equation of circle :
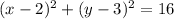
Now, In order to say that circle intersect y-axis , we have x=0 , Let's find out value of y for this:
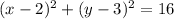
⇒

⇒
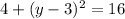
⇒

⇒
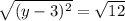
⇒

⇒
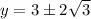
Therefore circle
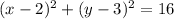 intersect y-axis at two points
intersect y-axis at two points
 and
and
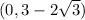 . And so statement is true!
. And so statement is true!