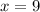Question

find x if the distance between points L and M is 15 and point M is located in the first quadrant. L=(-6,2) M=(x,2)​

Answer 1

Therefore,

`x = 9`

Explanation:

Given:

Let,

point L( x₁ , y₁) ≡ ( -6 , 2)

point M( x₂ , y₂ )≡ (x , 2)

l(AB) = 15 units (distance between points L and M)

To Find:

x = ?

Solution:

Distance formula between Two points is given as

`l(LM)^(2) = (x_(2)-x_(1))^(2)+(y_(2)-y_(1))^(2)`

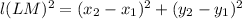
Substituting the values we get

`15^(2)=(x--6)^(2)+(2-2)^(2)\\\\225=(x+6)^(2)`

Square Rooting we get

`(x+6)=\pm √(225)=\pm 15\\\\x+6 = 15\ or\ x+6 = -15\\\\x= 9\ or\ x = -21`

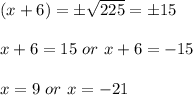
As point M is located in the first quadrant

x coordinate and y coordinate are positive

So x = -21 DISCARDED

Hence,

`x = 9`

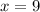
Therefore,

`x = 9`