Answer:
B) Since (-3,-2) does not satisfy both equations, it is not a solution to the
system.
Explanation:
The given system of equations is:
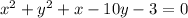
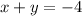
If (-3,-2) is a solution, then it must satisfy both equations:
Let us substitute into the first equation to get:
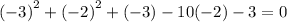
Evaluate the exponents;
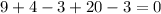
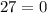
This is not true
Also when we substitute into the scond equation, we get;
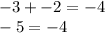
This is also false.
Therefore the point is not a solution.