Question

A water tank is in the shape of a right circular cone with height 18 ft and radius 12 ft at the top. If it is filled with water to a depth of 15 ft, find the work done in pumping all of the water over the top of the tank.

Answer 1

210,600πft-lb

Step-by-step explanation:

Force is a function F(x) of position x then in moving from

x = a to x= b

Work done =
`\int\limits^b_a {Fx} \, dx`

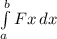
Consider a water tank conical in shape

we will make small horizontal section of the water at depth h and thickness dh and also assume radius at depth h is w

we will have ,

`(w)/(12) = ((18-h))/(18) \\w = (2)/(3) (18-h)a`

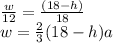
weight of slice under construction

weight = volume × density × gravitational constant

`weight = \pi * w^2 * dh * 62.4\\= (62.4\pi w^2dh)lb`

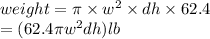
Now we can find work done

`W = \int\limits \, dw\\`

`\int\limits^(18)_(3) {(62.4\pi } \, dx w^2dh)h\\= 62\pi (4)/(9) \int\limits^(18)_(3) {(18-h)^2hdh} \,`

=
`62.4\pi *(4)/(9) ((324)/(2) h^2-(36)/(3) + (h^4)/(4))^1^8_3`

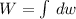
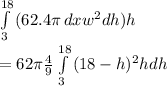
= 210,600πft-lb