Answer:
P(X=17) = 0.3002 .
Explanation:
We are given that the random variable X has a binomial distribution with the given probability of obtaining a success.
The above situation can be represented through Binomial distribution;
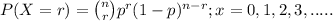
where, n = number of trials (samples) taken = 18
r = number of success = 17
p = probability of success which in our question is given as 0.9 .
So, X ~

We have to find the probability of P(X = 17);
P(X = 17) =
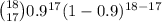
=
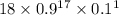 {
{
 }
}
= 0.3002
Therefore, P(X=17) = 0.3002 .