Answer:
No, there is not enough evidence at the 0.02 level to support the manager's claim.
Explanation:
We are given that the company's promotional literature states that 71% of the chips fail in the first 1000 hours of their use. Also, a sample of 1400 computer chips revealed that 69% of the chips fail in the first 1000 hours of their use.
And the quality control manager wants to test the claim that the actual percentage that fail is different from the stated percentage, i.e;
Null Hypothesis,
 : p = 0.71 {means that the actual percentage that fail is same as the stated percentage}
: p = 0.71 {means that the actual percentage that fail is same as the stated percentage}
Alternate Hypothesis,
 : p
: p
 0.71 {means that the actual percentage that fail is different from the stated percentage}
0.71 {means that the actual percentage that fail is different from the stated percentage}
The test statistics we will use here is;
T.S. =
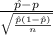 ~ N(0,1)
~ N(0,1)
where, p = actual percentage of chip fail = 0.71
 = percentage of chip failed in a sample of 1400 chips = 0.69
= percentage of chip failed in a sample of 1400 chips = 0.69
n = sample size = 1400
So, Test statistics =
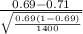
= -1.620
Now, at 0.02 significance level, the z table gives critical value of -2.3263 to 2.3263. Since our test statistics lie in the range of critical values which means it doesn't lie in the rejection region, so we have sufficient evidence to accept null hypothesis.
Therefore, we conclude that the actual percentage that fail is same as the stated percentage and the manager's claim is not supported.