Question

Machine A and Machine B can produce 1 widget in 3 hours working together at their respective constant rates. If Machine A’s speed were doubled, the two machines could produce 1 widget in 2 hours working together at their respective rates. How many hours does it currently take Machine A to produce 1 widget on its own?

Answer 1

`\boldsymbol{\mathbf{Answer}}`

`\boldsymbol{\mathbf{Machine \, A \,will\, take \,6 \,hours\, to \,produce\, 1 \,widget \,on\, its\, own.}}`

`\boldsymbol{\mathbf{Step-by-step \,explanation:}}`

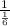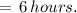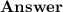
Let,

performance rate of machine A is x widget per hour.

performance rate of machine A is y widget per hour.

As given, Machine A and Machine B can produce 1 widget in 3 hours working together.

I.e mathemetically,

`\boldsymbol{x + y=(1)/(3)......(1)}`

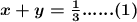
lly for second statement, Machine A’s speed were doubled, the two machines could produce 1 widget in 2 hours working together.

i.e mathematically,

`\boldsymbol{2x + y=(1)/(2)......(2)}`

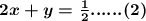
Substact equation (1) in (2)

`x + y=(1)/(3)`

`-2x + y=(1)/(2)`

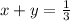
Resultant equation will be,

`-x=(-1)/(6)`

`\boldsymbol{x = (1)/(6)}`

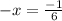
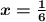
Performance rate of machine A is \frac{1}{6} widget per hour.

what is time Machine A will take to produce 1 widget on its own.

i.e =
`(1)/((1)/(6))`

`\boldsymbol\mathbf{{=\, 6 \,hours.}}`