Question

Find two numbers, if

Their sum is − 1/3 and their difference is 18

Answer 1

Let the two numbers be
`x,y`.

We have

`\begin{cases}x+y=-(1)/(3)\\x-y=18\end{cases}`

From the second equation, we derive
`x=18+y`

Plugging this value in the first equation, we have

`18+y+y=-(1)/(3) \iff 2y=-(1)/(3)-18\iff 2y=-(55)/(3) \iff y=-(55)/(6)`

And we derive

`x=18+y=18-(55)/(6)=(53)/(6)`

Answer 2

The two numbers are: -9.17 and 8.83

Explanation:

Let the two numbers represent 'x' and 'y'

Their sum is − 1/3 ==> x + y = -1/3 .................(eqn 1)

Their difference is 18 ==> x − y = 18 ....................(eqn 2)

from equation 2,

x = 18 + y

therefore, substitute for 'x' in (eqn 1) to get y

(18+y) + y = -1/3

18 + 2y = -1/3

2y = -1/3 − 18

2y = -
`18(1)/(3)`

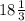
2y = - 55/3

y = (-55/3) / 2

y = -55/3 x 1/2

y = -55/6 = -9.17

Substitute for 'y' in either equation

picking (eqn 2)

x − (-9.17) = 18

x + 9.17 = 18

x = 18 − 9.17

x = 8.83