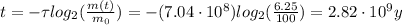Answer:

Step-by-step explanation:
A radioactive isotope is an isotope that undergoes nuclear decay, breaking apart into a smaller nucleus and emitting radiation during the process.
The half-life of an isotope is the amount of time it takes for a certain quantity of a radioactive isotope to halve.
For a radioactive isotope, the amount of substance left after a certain time t is:
 (1)
(1)
where
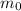 is the mass of the substance at time t = 0
is the mass of the substance at time t = 0
m(t) is the mass of the substance at time t
 is the half-life of the isotope
is the half-life of the isotope
In this problem, the isotope is uranium-235, which has a half-life of
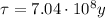
We also know that the amount of uranium left in the rock sample is 6.25% of its original value, this means that
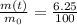
Substituting into (1) and solving for t, we can find how much time has passed: