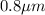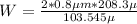Answer:

Step-by-step explanation:
the transistor In saturation drain current region is given by:
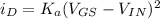
Making
 the subject of the formula; we have:
the subject of the formula; we have:
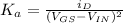
where;
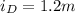
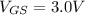

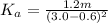

Also;

where:
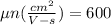

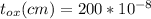
substituting our values; we have:
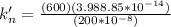
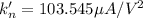
Finally, the width can be calculated by using the formula:
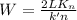
where;
L =