Answer:
The unit cell edge length of the alloy is 0.3112 nm
Step-by-step explanation:
Given;
density of chromium, Cr= 7.19 g/cm³
atomic weight of chromium, Cr = 52.00 g/mol
density of tantalum, Ta = 16.6 g/cm³
atomic weight of tantalum, Ta = 180.95 g/mol
Step 1: determine the average density of the atoms;
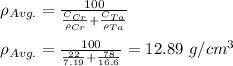
Step 2: determine the average atomic weight;
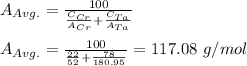
Step 3: determine the cubic volume of the atoms;
there are 2 atoms per unit cell of a body centered cubic structure.
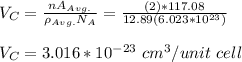
Step 4: determine the unit cell edge length;
Vc = a³
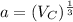
Where;
a is the edge length
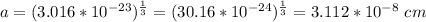
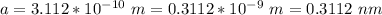
Therefore, the unit cell edge length of the alloy is 0.3112 nm