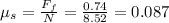Question

A curve of radius 151 m is banked at an angle of 11°. An 838-kg car negotiates the curve at 86 km/h without skidding. Neglect the effects of air drag and rolling friction. Find the following.

(a) the normal force exerted by the pavement on the tires
kN ?

(b) the frictional force exerted by the pavement on the tires
kN ?

(c) the minimum coefficient of static friction between the pavement and the tires?

Answer 1

a) 8.52 kN

b) 0.74 kN

c) 0.087

Step-by-step explanation:

a)

There are 3 forces acting on the car on the banked curve:

- The weight of the car,
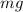`mg`, vertically downward

- The normal force of the pavement on the tires, N, upward perpendicular to the road

- The force of friction,
`F_f`, down along the road

Resolving the 3 forces along two perpendicular directions (horizontal and vertical), we obtain the equations of motions:

x- direction:

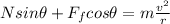`N sin \theta +F_f cos \theta = m (v^2)/(r)` (1)

y- direction:

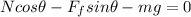`Ncos \theta -F_f sin \theta-mg =0` (2)

where

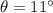`\theta=11^(\circ)` is the angle of the ramp

`F_f` is the force of friction

m = 838 kg is the mass of the car

r = 151 m is the radius of the curve

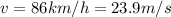`v=86 km/h =23.9 m/s` is the speed of the car

Solving eq.(2) for Ff and substituting into eq.(1), we can find the normal force:

From (2):

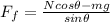`F_f=(Ncos \theta-mg)/(sin \theta)` (3)

Substituting into (1) and re-arranging,

`N sin \theta +(N cos \theta -mg)/(sin \theta) cos \theta = m (v^2)/(r)\\N(sin \theta+(cos^2 \theta)/(sin \theta))=m(v^2)/(r)+mg(cos \theta)/(sin \theta)\\N=((mv^2)/(r)+mg (cos \theta)/(sin \theta))/(sin \theta + (cos^2\theta)/(sin \theta))=8518.3 N = 8.52 kN`

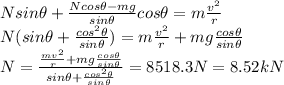
b)

The frictional force between the pavement and the tires,
`F_f`, can be found by using eq.(3) derived in part a):

`F_f=(Ncos \theta-mg)/(sin \theta)`

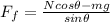
where we have:

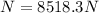`N=8518.3 N` is the normal force

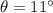`\theta=11^(\circ)` is the angle of the ramp

m = 838 kg is the mass of the car

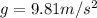`g=9.81 m/s^2` is the acceleration due to gravity

Substituting the values, we find:

`F_f=((8518.3)cos 11^(\circ)-(838)(9.81))/(sin 11^(\circ))=739.0 N = 0.74 kN`

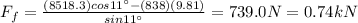
c)

The force of friction between the road and the tires can be rewritten as

`F_f=\mu_s N`

where

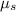`\mu_s` is the coefficient of static friction

N is the normal force exerted by the road on the car

In this problem, we know that

N = 8.52 kN is the normal force

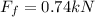`F_f=0.74 kN` is the frictional force

Therefore, the minimum coefficient of static friction between the pavement and the tires is:

`\mu_s = (F_f)/(N)=(0.74)/(8.52)=0.087`