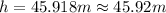Question

Read the scenario and solve these two problems.

When traveling at top speed, a roller coaster train with a mass of 12,000 kg has a velocity of 30 m/s. The kinetic
energy of the train at top speed is
Given this kinetic energy, what is the tallest hill this roller coaster train can reach the top of?
The train can climb a hill that is m high.

Answer 1

1 c 2a

Step-by-step explanation:

Answer 2

Answers:

a) 5400000 J

b) 45.92 m

Step-by-step explanation:

a) The kinetic energy
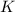`K` of an object is given by:

`K=(1)/(2)mV^(2)`

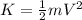
Where:

`m=12000 kg` is the mass of the train

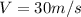`V=30 m/s` is the speed of the train

Solving the equation:

`K=(1)/(2)(12000 kg)(30 m/s)^(2)`

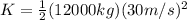
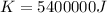`K=5400000 J` This is the train's kinetic energy at its top speed

b) Now, according to the Conservation of Energy Law, the total initial energy is equal to the total final energy:

`E_(i)=E_(f)`

`K_(i)+P_(i)=K_(f)+P_(f)`

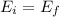
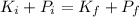
Where:

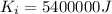`K_(i)=5400000 J` is the train's initial kinetic energy

`P_(i)=0 J` is the train's initial potential energy

`K_(f)=0 J` is the train's final kinetic energy

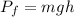`P_(f)=mgh` is the train's final potential energy, where
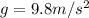`g=9.8 m/s^(2)` is the acceleration due gravity and
`h` is the height.

Rewriting the equation with the given values:

`5400000 J=(12000 kg)(9.8 m/s^(2))h`

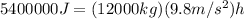
Finding
`h`:

`h=45.918 m \approx 45.92 m`